For a graph
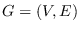
, a labeling
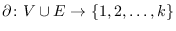
is called an edge irregular total

-labeling of
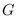
if the weights of any two different edges are
distinct, where the weight of the edge
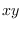
under
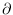
is
defined to be
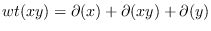
. The
total edge irregularity strength

of
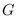
is the
minimum

for which
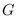
has an edge irregular total

-labeling.
Al-Mushayt et al. “prove" that
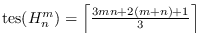
for the hexagonal grid graph
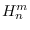
, but the labeling they
constructed is actually not a total
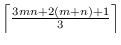
-labeling. In this
paper, we first describe a correct edge irregular total
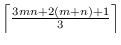
-labeling of
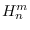
for any
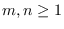
, and so show that
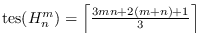
.
Moreover, we determine the exact value of the total edge
irregularity strength for a more general hexagonal grid graph
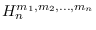
by giving an edge irregular total
tes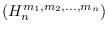
-labeling, where
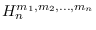
consists of

columns of hexagons and
has
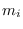
hexagons in the
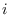
-th column,
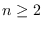
, and
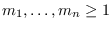
.